Typically your intestine emotions lead you astray—notably in arithmetic, during which one consistently comes throughout outcomes that appear inconceivable. For instance, infinity does not all the time equal infinity, and tortoises might outpace human athletes—no less than from a sure mathematical standpoint.
There are additionally many situations that seem contradictory at first look (or second or third). These paradoxes could be defined, nonetheless. They don’t seem to be errors however somewhat reminders that we must always not rely too closely on our instinct in arithmetic. Listed below are three of the strangest paradoxes within the subject.
Hilbert’s Resort
On supporting science journalism
For those who’re having fun with this text, take into account supporting our award-winning journalism by subscribing. By buying a subscription you might be serving to to make sure the way forward for impactful tales in regards to the discoveries and concepts shaping our world as we speak.
Think about you might be touring to a metropolis and have forgotten to guide a room beforehand. Thankfully, you come throughout a fantastic lodge named after the well-known mathematician David Hilbert, whose work you drastically respect. You step as much as reception and see that the lodge has an infinite variety of rooms: the room numbers correspond to the pure numbers 1, 2, 3, 4, … with out ever coming to an finish.
The receptionist tells you that the lodge is absolutely booked, nonetheless. However you recognize your approach round math, so that you don’t let your self be fobbed off so simply. a trick that can enable you—and all the opposite limitless company—to discover a room, too. You counsel to the receptionist that every visitor transfer to the room numbered one increased than their present lodging. So the individual from room 1 goes to room 2, the individual from room 2 to room 3, and so forth.
As a result of Hilbert’s lodge has a limiteless variety of rooms out there, even when absolutely booked, there may be nonetheless room for extra company. And that’s not simply the case for one individual: they may have introduced an entire busload of people that additionally wished a room. On this case, the lodge company must transfer not only one however a number of room numbers away.
It will get even stranger. Even in the event you deliver an infinite variety of individuals to Hilbert’s lodge, you possibly can nonetheless accommodate them within the absolutely booked lodge. To do that, the visitor in room 1 must transfer to room 2, the visitor in room 2 to room 4, the visitor in room 3 to room 6, and so forth. As every individual strikes right into a room with a quantity that’s twice their present room quantity, an infinite variety of odd-numbered rooms turn out to be out there.
By shifting every visitor right into a room with a quantity twice their present one, there may be house for an infinite variety of extra individuals.
Jan Beránek/Wikimedia (CC BY-SA 4.0), restyled by Amanda Montañez
German mathematician David Hilbert offered this supposed paradox throughout a 1925 lecture on infinity. The instance illustrates how not all ideas could be transferred from finite to infinite instances: the statements “every room is occupied” and “the hotel cannot take any more guests” are synonymous in the actual world—however not in a world with infinities.
The Birthday Paradox
The subsequent paradox is extra acquainted to many. After I was at college, it was not unusual for a number of of my classmates to have their birthday on the identical day. Actually, I additionally shared a birthday with a classmate. At first, this looks as if an enormous coincidence. In spite of everything, a yr has three hundred and sixty five days (or 366 in leap years, however we’ll ignore that for the sake of simplicity), and a college class consists of round 20 to 30 college students. Our intestine feeling subsequently tells us that it’s unlikely that two youngsters have been born on the identical day.
However that’s not true. Actually, the chance that two individuals in a bunch of 23 have a birthday on the identical day is greater than 50 p.c. To higher perceive this, it helps to look not on the variety of individuals however on the variety of pairs of individuals. From 23 individuals, (23 x 22) / 2 = 253 pairs could be fashioned—and this quantity exceeds half of all the times in a yr. If we take a look at the chance that one of many pupils in a college class of 23 was born on a specific date, nonetheless, the chance is barely 1- ((365-1) /365)^23=6.1 p.c.
The birthday paradox subsequently arises from the truth that taking a look at pairs of scholars provides you a better variety of potentialities than in the event you solely take a look at people.
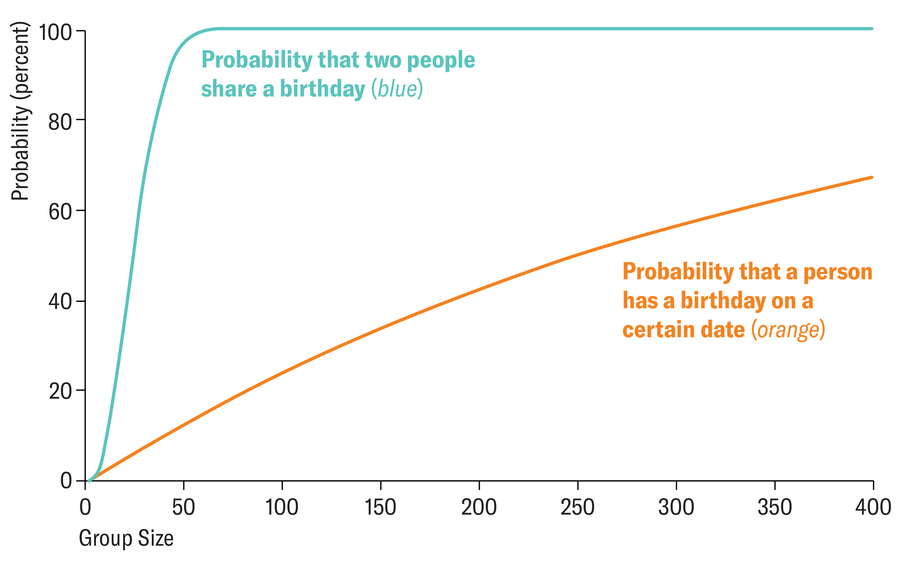
The blue line signifies the chance that two individuals from a bunch (group measurement famous on the x axis) have the identical birthday. The orange line corresponds to the chance that an individual has a birthday on a sure date.
Toobaz/Wikimedia (CC BY-SA 4.0), restyled by Amanda Montañez
This reality has tangible results in cryptography, for instance. If you wish to signal a digital contract, for instance, “hash functions” are used: The doc is transformed into a personality string (a “hash”) of a hard and fast size when it’s signed. If even the smallest change is made to the unique doc, the hash that’s fashioned from it’s fully totally different. By maintaining their hash, the signatory can show what they initially signed—making the method tamper-proof. There may be an especially low chance that two fully totally different paperwork will generate one and the identical hash, nonetheless, and that poses a safety danger.
As a rule, the size of the hash operate is chosen in order that such “collisions” (the place two totally different information information produce the identical hash) are extraordinarily uncommon. A hacker can perform a “birthday attack,” nonetheless: they’ll generate many various paperwork and examine their hash features in pairs—simply as a instructor compares the birthdays of classmates as a substitute of specializing in a particular date and a single pupil.
In observe, a birthday assault might appear like this: I first create two contracts, V1 and V2. V1 is a good contract, however V2 has wording that’s in my favor. Then I alter each contracts in numerous locations: I add areas, tabs and line breaks to create variations of V1 and V2. These adjustments are nearly invisible to a reader, however they drastically change the hash operate of the paperwork.
If I examine the person hash features of the modified contracts V1 and V2 in pairs, I’ll discover a matching hash way more shortly than if I particularly attempt to reproduce a specific hash (reminiscent of that of V1). If I discover a matching pair of V′1 and V′2, I can provide the contract V′1 to signal however declare afterward that you just signed V′2. As a result of each generate the identical hash, the fraud can’t be detected by digital signature software program.
Russell’s Antinomy
British thinker Bertrand Russell formulated a paradox in 1901 typically referred to as Russell’s antinomy—a time period for a press release that describes two seemingly contradictory concepts. Not like Hilbert’s lodge and the birthday paradox, Russell’s antinomy isn’t a outcome that merely eludes our instinct. It contradicts the foundations of logic per se. The antinomy produces statements that may be neither false nor true.
There are a number of examples that may illustrate Russell’s antinomy, however one well-known case is the “barber paradox.” Suppose a barber shaves all the boys on the town who don’t shave themselves—and solely these males. Does the barber shave himself? If he shaves himself, then he now not belongs to the group of people that don’t shave themselves. But when he doesn’t shave himself, then, by definition, he must shave himself (as a result of all residents who don’t shave themselves go to him).
This downside arises due to poorly outlined units. On the time that Russell offered his antinomy, a set typically referred to a set of issues: The pure numbers, for instance, kind a set, as does the set of all inhabitants that don’t shave themselves. This additionally permits units to include themselves or confer with themselves as an entire—and these properties result in contradictions. This antinomy subsequently led to the top of what mathematicians name “naive set theory.”
The muse of arithmetic continues to depend on set concept. However units on this assemble are now not mere collections and as a substitute should fulfill sure situations. For instance, units should be composed of already present units and should not confer with themselves. This guidelines out antinomies such because the barber paradox.
To place this in mathematical notation: individuals on the town who can develop a beard and are males kind a set M. That set consists of the boys who shave themselves and those that don’t. Subsequent, the set C consists of all of the barber’s clients. To kind C, you need to comply with the foundations of contemporary set concept: If the barber is a person with a beard, or a part of M, then the set of consumers can’t be outlined as “all male residents who do not shave themselves”—as a result of on this case the definition would confer with itself with each the barber and the purchasers as a part of M. Set concept merely doesn’t allow such a definition. But when the barber isn’t a part of M—for example, if the barber is a girl or unable to develop a beard—then the definition is permitted.
We are able to now breathe a sigh of reduction: the paradoxes have been solved, and arithmetic isn’t doomed to failure. There isn’t a assure that the mathematical guidelines won’t in some unspecified time in the future produce a unresolvable contradiction, nonetheless. Logician Kurt Gödel proved this within the Thirties—and in doing so made it clear that there isn’t a certainty that arithmetic will work endlessly in a self-contained approach. One of the best we will do is hope that an unsolvable contradiction by no means arises.
This text initially appeared in Spektrum der Wissenschaft and was reproduced with permission.

